Lesson Outline and Objective
Algebra tiles are a powerful tool for helping students make the connection between symbolic manipulation and geometrical representation using area models. In this activity, students can explore completing the square using the Polypad Algebra tiles, and get a sense of why the process is called “completing the square”.
Students will understand how to move between the forms and for a quadratic expression.
Warm Up
Optional Review of Area Model of Multiplication
If your students could benefit from a review of the area model of multiplication, consider solving 27 x 15 in three different ways using this Polypad canvas:

This can also serve as a good review of multiplication of negative numbers. This Polypad canvas shows the solutions:

Spot the Squares
Start by reminding students how to expand expressions of the form using an example such as which can be represented using algebra tiles using this Polypad canvas:

Then give students this list of expressions. Can they spot which can be written as ?
Students may wish to explore using the Polypad algebra tiles to start off with, but by thinking about the structure of the squares that they make, students may be able to answer some of the questions without needing to create the diagrams. It might be worth sharing as a class any strategies that students used to help them decide whether an expression could be written as a square or not.
Solutions
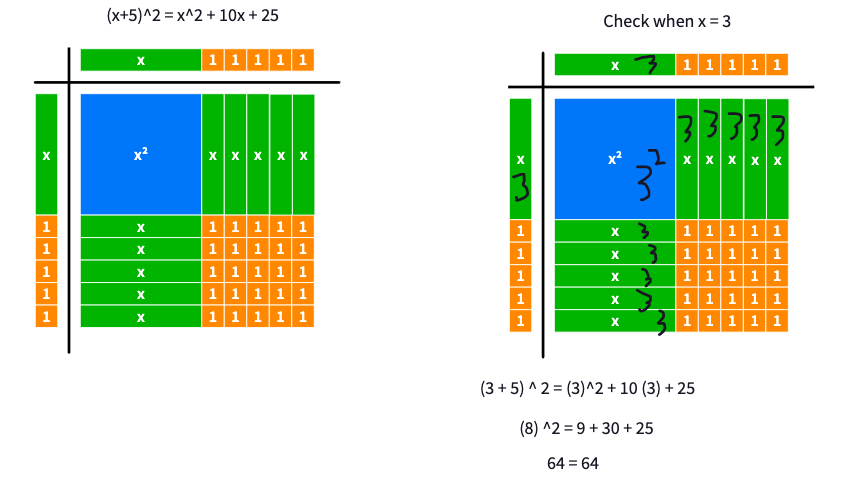
If your students could benefit from a reminder of equivalent expressions, consider substituting in a value for x in one of the solutions above to show that the two expressions are equal (at least for the chosen value of x). To show this visually on Polypad, you could use the pen tool and the algebra tiles to show this check for :
Main Activity
Share this Polypad canvas and allow students time to work on forming squares with the tiles provided:
After giving students some time to work,, discuss the algebraic representations of their findings.
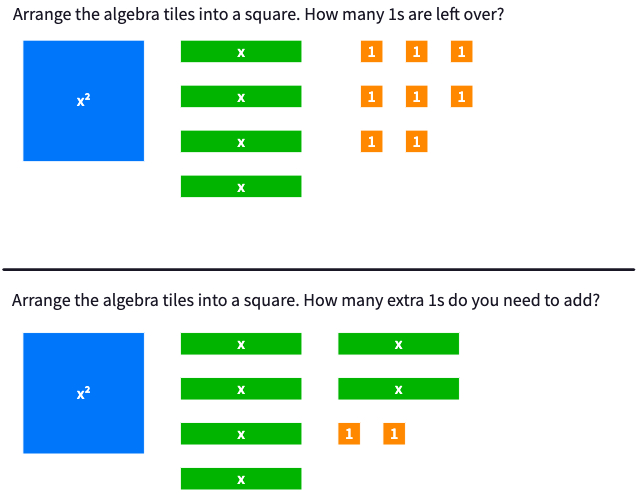
In the first example, , there will be tiles left over:

Discuss with students that this pictures shows that by itself cannot equal a square. comes close to equalling , but there were four leftover from the 8. So,
For the second example, , there will be gaps that need to be filled:
One way to manage filling in the gap is to add pairs of 1 and -1 tiles (which keeps the expression the same), giving:
This shows that .
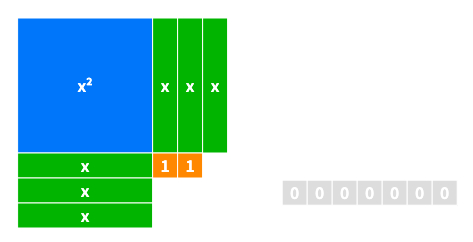
You can show your students that the +1 and -1 tiles cancel out to make 0’s by dragging them on top of each other. This shows that you’re not changing the value of the expression on the canvas:
Now, take apart the 0 tiles and move use some of them to create a square:
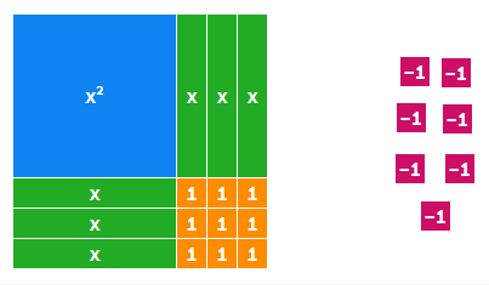
Conclude that
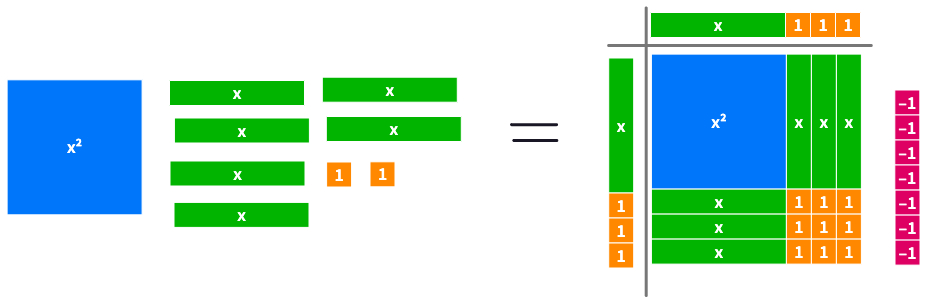
Given the ease of adding tiles to the canvas, some students may think just adding 7 tiles to the diagram “completes the square.” So, they may incorrectly go from the first image below to the second image:

Clarify with students that the first picture represents and the second picture represents . Show that these are NOT equivalent by asking students for a value of x. Use that value of x to evaluate each expression. Discuss how adding 7 to the diagram was changing the value of the expression. In order to add 7 of the 1 tiles without changing the overall value of the expression, we must also subtract 7 (or add 7 of the -1 tiles) so the overall impact is 0.
Now give students some time to explore completing the square. Here are some expressions they could try:
The first is chosen to be similar to the examples they have already seen. The second has a negative constant term, and the third introduces a negative coefficient of x so they will need to think carefully about positive and negative numbers. The fourth would require cutting up the algebra tiles as the coefficient of x is odd, so this could be a good focus for discussion at the end of the lesson.
Students who have already learned how to factorize quadratics will know that not every quadratic can be factorized, so it’s worth taking the time to discuss that it is always possible to express a quadratic expression of the form in the form , although p and q will not necessarily by whole numbers, as seen in the fourth example.
Towards the end of the lesson, it may be worthwhile to take some time to use a graphing program or graphing calculators to plot the graphs for all the examples ( , and so on). Doing so can help explore the relationship between the coordinates of the vertex of the parabola and the values of p and q in the completed square form of each expression.
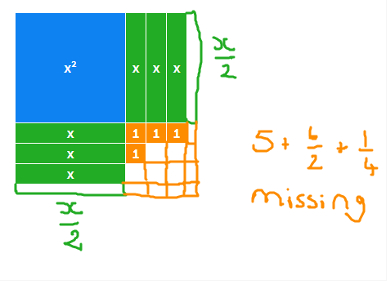
Possible Solutions
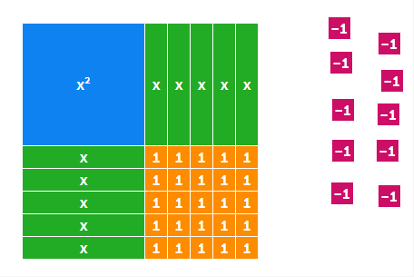
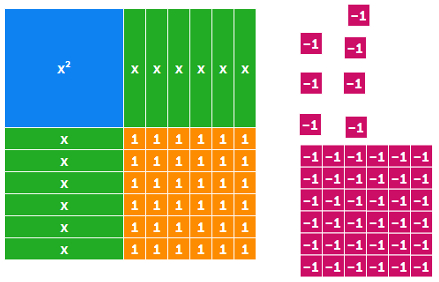
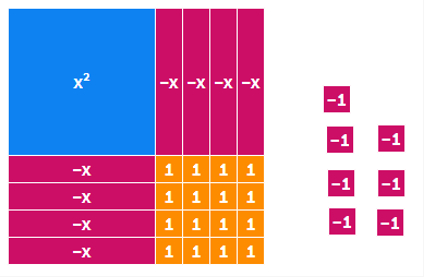
As this last example uses fractions, students can’t represent it directly using algebra tiles but may wish to annotate using the pen tool to show the parts of their answer:
Support and Extension
Completing the square is considered a tricky topic. Using the algebra tiles can really help to make it more concrete, so the best way to support students who are struggling is to give them lots of time to become familiar with algebra tiles, perhaps by exploring expansion and factorizing before moving on to completing the square. It is worth spending plenty of time on squaring brackets so that completing the square feels like just one more step – trying to make a square from the and the pieces and then seeing what must be added or taken away to complete the square!
A nice extension to this activity is to invite students to think about how they could use completing the square to help them solve quadratic equations. As with all explorations of quadratics, it is worthwhile to encourage students to move between algebraic and graphical representations and inviting them to explain any relationships they notice so that they start to develop a better understanding of how features of an algebraic representation relate to features of the graph.