Overview and Objective
In this lesson, students solve systems of two linear equations in two variables using the balance scale on Polypad. They explore that the solution is an ordered pair that satisfies each equation independently. At the end of the lesson, they create their systems of equations for classmates to solve.
Warm-Up
Share this Balance Canvas with students and invite them to work on finding the values for each object. Then, take some time to discuss their findings, perhaps using the following prompts for discussion:
- How many variables are there? How many equations?
- Which strategies did they use to solve the equations? Did they try to eliminate one of the variables? Did they substitute the values?
- Which equations were easy to start with?
- How can we express these equations algebraically?
Discuss that sometimes equations that have more than one unknown can have infinitely many solutions. Therefore, to solve an equation with two or more variables, other equations are needed to be used alongside it. That way, it is possible to find the values that solve equations at the same time. These are known as simultaneous equations or systems of equations.
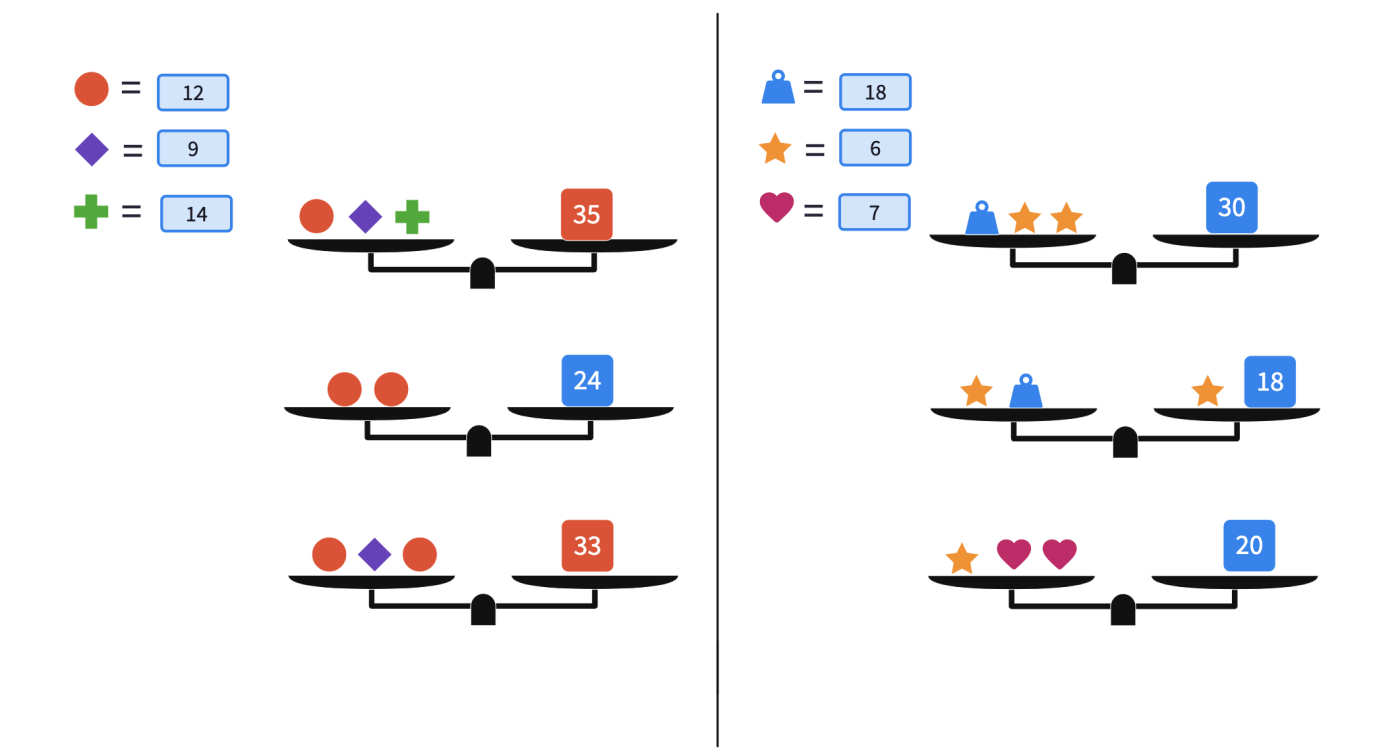
Main Activity
Share the first example with the students using the "System of Equations 1" canvas.
Ask them to express the equations using numbers and variables as well. Then, share some student work and discuss the strategies that students used to find the value of each variable.

If they solve the equations individually, there are infinitely many solutions; you can use the table tool to list some integer values that satisfy each equation. Here, students can see the ordered pair satisfies both the equations. Therefore it is the solution to this system of equations.
If they already knew about graphing the equations, you can graph them on the coordinate plane and ask about the intersection point. The students will explore the coordinates of the intersection point shows the solution of the equation system too.
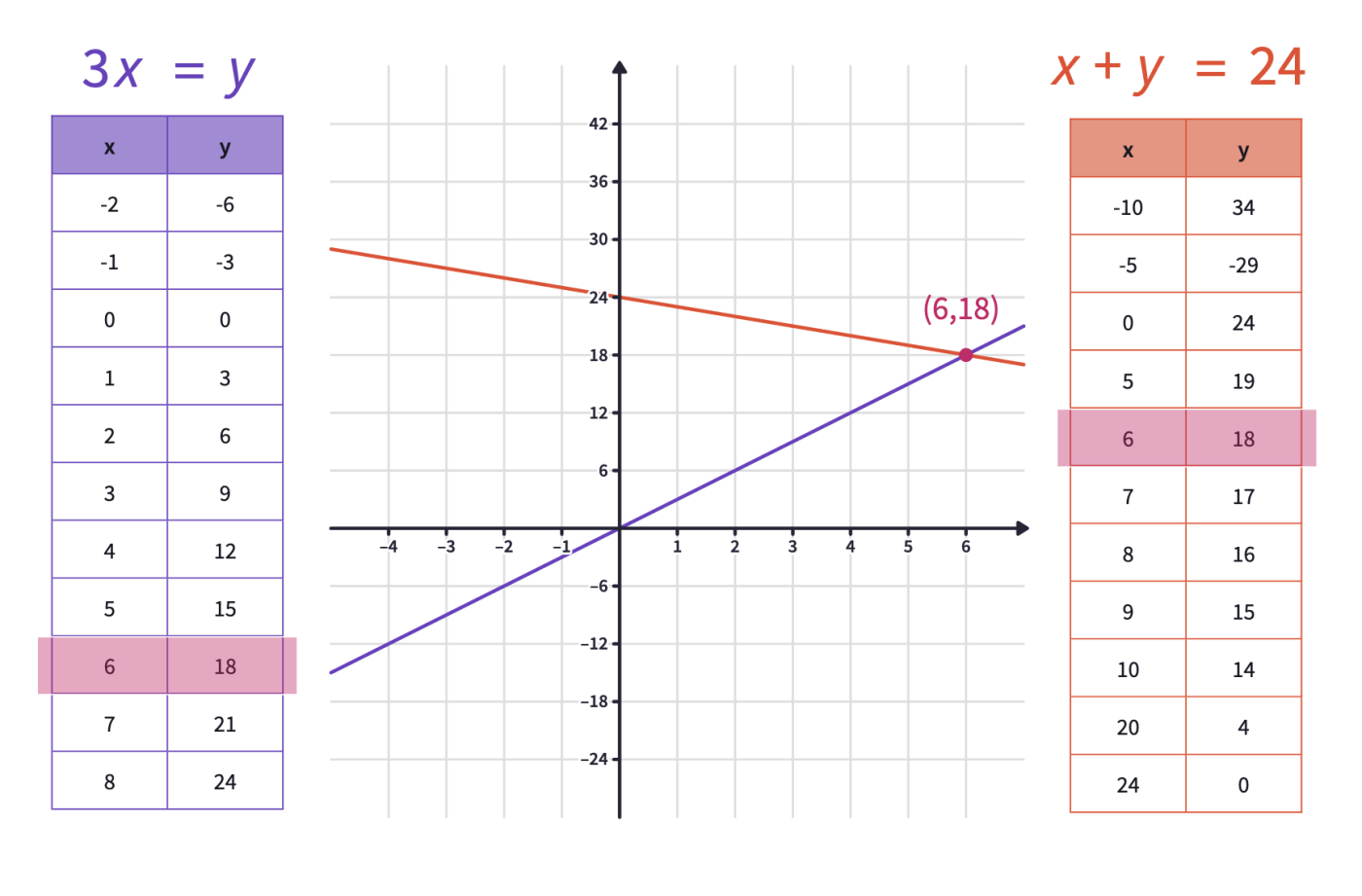
Ask about the possible difficulties of these methods to solve the system of equations.
If they did not use the substitution idea already, demonstrate the method by replacing the weight with three hearths. Discuss if the balance stayed the same or changed.
You may also show the same steps algebraically.
Once they find the value of one of the variables, they can substitute its value in either equation to find the other variable.
For the Second Example, share the "System of Equations 2" canvas. Let them work on the equations and share their strategies.
Ask students to write the equations algebraically again.
Then let them draw the graphs of the equations and read the intersection point. Invite students who had a different strategy to share how they find the value of the variables.
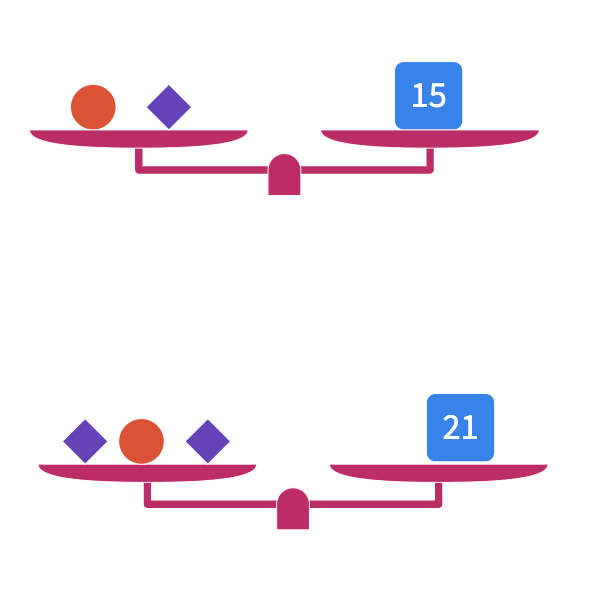
Discuss the strategy of adding or subtracting the equations. Does the balance stay the same or change? Let them try and share their results. Ask if this method can also be helpful to solve the simultaneous equations.
When we add the equation side by side, the balance did not change.
When we get the difference of the equations, the balance stayed the same and gave the value of one of the variables directly. As if the balance changes when you multiply both sides by the same number or not? You may use their findings to create a set of possible strategies to solve the equations algebraically. You can name the methods as and here.
For the third example, share the "System of Equations 3" canvas.
Ask them to solve the system as many methods as they can.
Invite students to share their work and discuss which approaches they found most useful when answering this question.
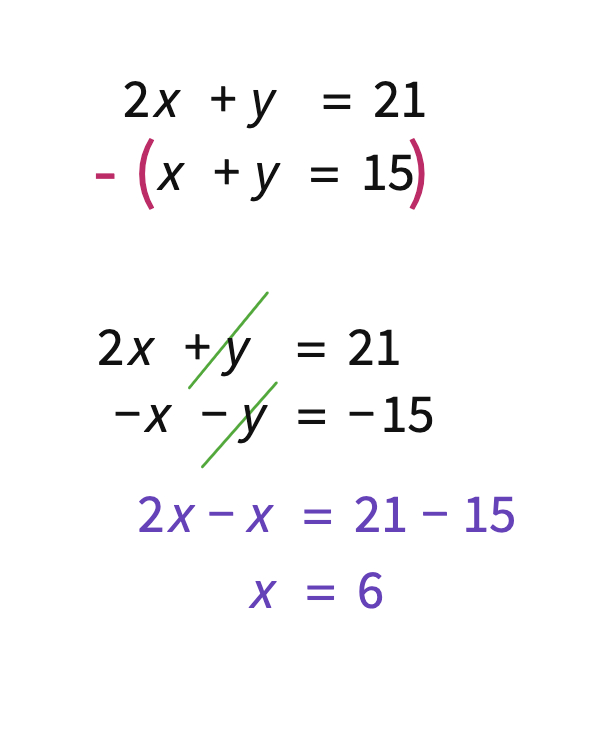

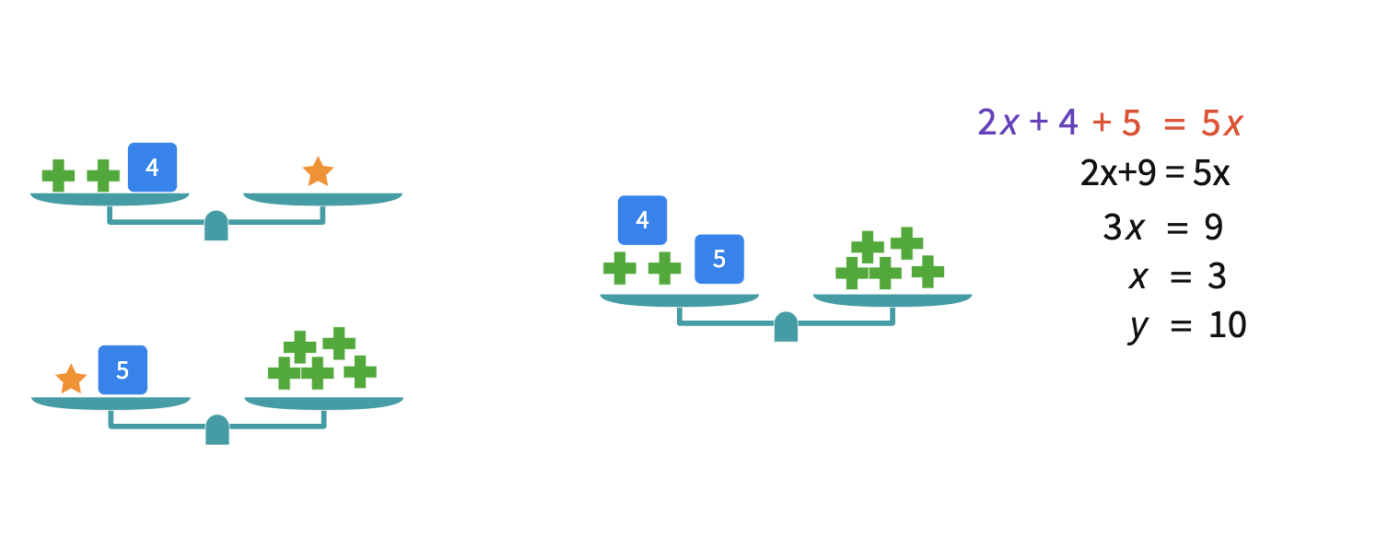
Clarify with the students that they found the unknowns in the first warm-up puzzle by substituting variables with numbers. Sometimes one may need to substitute a variable with another variable or the combination of a variable and a number.
Closure
To close the lesson, consider having students work in pairs and ask each student to create a system of equations on the balance scale. You may show this short demonstration as a How-to video if needed. After they all created their simultaneous equation, let them solve each other's equations.
- Place two scales on the canvas.
- Pick two different objects as your variables. Set their values by balancing the scales.
- Create two distinct equations. When you have created the correct equations, the scale will be automatically balanced. Click here to learn more about using the Balance Scale.
Support and Extension
For students ready for additional extension in this lesson, consider asking the Balance Scale puzzle. For students needing additional support with these ideas, give more examples with easier equations.
Polypads for This Lesson
To assign these to your classes in Mathigon, save a copy to your Mathigon account. Click here to learn how to share Polypads with students and how to view their work.